3 essential components of math motivation

Last year, Renaissance released Trip Steps for Mathematics, which are essential math skills that are also disproportionately difficult for students to learn. The Trip Steps begin in pre-K, with the skill Count on with numbers 1 to 10, and continue through Algebra 1, with the skill Complete the square in a quadratic expression to find the maximum or minimum value of the function it defines. In between, students encounter challenging skills in multiple domains, from Whole Number Operations to Fraction Concepts to Geometry and Measurement. Identifying the challenges that students will face from counting to 10 to defining the function supports their learning journey, but more is required. The will, or motivation, to do the work and tackle the setbacks is essential.

In an earlier blog, a colleague and I describe Renaissance’s process for identifying Trip Steps for Mathematics. In this blog, I’ll continue the conversation by addressing a question I often receive from educators when I share Trip Steps: How can we motivate students to learn these challenging skills—especially students who already struggle with math?

It’s an important question. Perhaps math motivation can be thought of as the instructional counterpoint to a Trip Step. Just as a Trip Step is disproportionately difficult for students to learn, motivation can be a disproportionately difficult instructional practice for teachers to implement. In the same way that students dig deeper into a challenging Trip Step, teachers seek resources, advice, and proven strategies to motivate students. So, how do we build math confidence and stamina among all learners? In this blog, I’ll explore three key factors—executive, emotional, and environmental—at work in math motivation. I’ll also share strategies to inspire, motivate, and engage growing mathematicians in the new school year.

1. Executive function and mathematics

As Stanislas Dehaene (2011) explains, newborns are equipped with some degree of number sense. They’re able to distinguish two objects from three and three objects from four—although most show a preference for groups of three. At six months of age, children recognize groups of objects and even play by combining and separating them—a precursor to learning addition and subtraction (Dehaene, 2017). This is impressive, but they lack the ability to order numbers. In other words, three blocks intrigue them, but they have no concept of the number “3” or what this represents. Why not? Because ordinal competency (i.e., counting ability) depends on executive function—in particular, organizing, planning, and updating working memory. Between 6 and 15 months, however, children are continually updating their working memories to the point that ordinal competency—associating quantity with number—is well underway.

In fact, executive function rather than IQ supports counting ability, planning, organizing objects, and subitizing—which is the ability to immediately recognize the quantity of a group of objects without physically counting each one (Kroesbergen et al., 2009).

Motivation with executive function in mind

Why does this insight matter? Let’s return to the very first math Trip Step from pre-K: Count on with numbers 1 to 10. With our youngest learners, games focused on counting and subitizing build both math skill and executive function. Simply counting objects requires emerging mathematicians to keep track of the number of objects and the word(s) that describe numbers (e.g., three, four, five). Students who struggle with working memory—in this case, counting memory—benefit from simple counting games throughout the day and playing games with subitizing (Hutchison & Phillips, n.d.).

Intermediate students benefit when executive function is focused on organization, updating working memory, and flexibility. The well-known Principal’s Math Challenge offers a motivator focused on flexibility. Students work in pairs to create an expression for each number from 0–50, using only 1, 2, 3, and 4. All operations can be used, but each of the four numbers must be used—and used only once. Negative numbers are fair game, too. Here is one example: (4 × 3) ÷ (1+2) = 4. Try a few expressions to gauge your own executive level of flexibility in mathematical thinking.

2. Emotional sense of belonging and mathematics

In a previous blog, I explored two ways that students describe themselves as mathematicians. According to Kimball and Smith (2013), there is one key difference between students who achieve in math and those who don’t—entity orientation. Entity orientation is the belief that math ability is innate: you’re either born with the circuitry for math or you’re not, and nothing can change this. The opposite approach is known as incremental orientation. Students with incremental math orientations believe that math is difficult, but if they take it one skill at a time, they can learn it.

With this distinction in mind, let’s consider the most difficult math Trip Step. It’s found in grade 3: Find the area of a rectangle by multiplying side lengths. While this skill seems straightforward to adults, it’s extraordinarily difficult for many third graders to learn, given that multiplication is new, as is the idea of finding area by multiplying rather than by tiling. Yet we often forget this. With the best of intentions, we might begin a lesson on this skill by explaining that it’s easy: Just multiply! But as mathematician and author Jordan Ellenberg (2021) brilliantly explains, “When we say a lesson is ‘easy’ or ‘simple,’ and it manifestly isn’t, we are telling students that the difficulty isn’t with the mathematics, it’s with them. And they will believe us.”

Following Ellenberg’s advice, the stronger approach to teaching this Trip Step is to level with students. Here’s an example: “Today we’re going to find the area of a rectangle. This is a new skill, it is difficult, and you might get frustrated. But we will work as a team and support each other until everyone is successful. Then we can celebrate as a team.”

Motivation with emotional responses to mathematics in mind

Why do emotions matter? Students’ emotional responses to math instruction and experiences influence their attainment of math skills and their motivation to work through challenging concepts. In this regard, one of the most effective resources a student has is another student. Consider this Algebra 1 Trip Step: Determine the slope of a line given a graph, two points on the line, or a table of values. Rather than delivering a lecture on slope, educator Angela Cooper (2017) encourages her students to discuss this concept with each other, using the language they already have. Some students will speak of “straight” and “slanted” lines; others will talk about the “slope” or “regression.”

As students work together as a team, Cooper notices that—with scaffolding and support where needed—their language becomes increasingly precise: “By creating direct connections between what they already know (slant) and what they need to know (slope), they find that the latter is a much more precise and specific way to get at the concept they’re trying to describe.”

3. Environment and mathematics

The US Bureau of Labor Statistics describes mathematicians as people who analyze data to solve problems. For example, many neighborhood parks in the US either have installed, or are now installing, accessible ramps, swings, and play areas so that each child can feel included and share in the joy found outdoors. Can you imagine the problem-solving skills required to build a safe and accessible swing? Yet mathematicians solved this problem in order to meet a far bigger challenge: providing inclusive areas for all children to play. Their work, while difficult, led the way to accessible playgrounds.

In this case, solving problems of slope, area, circumference, velocity, and drag is essentially kind. Consider this grade 7 Trip Step that could be the foundation for eventually providing an inclusive experience for each child: Solve a problem involving the surface area or volume of a 3-dimensional object composed of cubes and/or right prisms.

Motivation with a kind math environment in mind

Why does a focus on kindness matter? As seventh graders and their teachers tackle this Trip Step, collaboration and encouragement can be motivating while also helping to build a kind math environment. Consider a story shared by Alan November (2018) about a sixth grader who was stumped by prime factorization. Her teacher encouraged peer tutoring, and—with support from her classmates—this student was finally on her way. Her teacher also encouraged students to create video tutorials, which were then posted on YouTube. Having learned prime factorization, our student decided to record a tutorial.

When November visited her classroom, he asked whether she’d seen the statistics. Her tutorial had been viewed more than 88,000 times by students all over the world. At this point, our formerly frustrated and struggling student said, “I have to go—I have to create more. I just found out the world needs me!”

These examples show us how the math environment can be focused on “math for good.” What students learn today can positively impact others in the future. The focus remains on math, but with a foundation that mathematicians solve problems that solve problems.

The power of real-world connections

Engaging in authentic math experiences requires mathematical flexibility rooted in executive function, a sense of purpose—or emotion—for the outcomes, and a healthy environment in which to work. However, as Craig Barton (2018) reminds us, engaging in authentic experiences is not the result of “taking the math outside; rather, it is the result of explicit, incremental, and monitored instruction that prepares students to solve problems that solve problems.”

For this reason, Renaissance’s Freckle for math program includes Inquiry Based Lessons that help students to deepen their conceptual understanding by applying math to real-world scenarios, such as planning a trip to the local library (which involves subtraction skills) or scuba diving in lakes and oceans (which focuses on decimals). The lessons include short videos, discussion questions, and group activities, with students then presenting their solution to the class and explaining their thinking.

Inquiry Based Lessons also involve cross-curricular connections. For example, the grade 3 lesson “Passing a Bill into Law” brings together mathematics and social studies content. The lesson is spread over several days, with students calculating how many votes a piece of legislation still needs in order to pass the US Congress. Over the course of the lesson, students encounter scenarios that require them to add and subtract four-digit numbers using multiple strategies, as shown in the screenshot below.

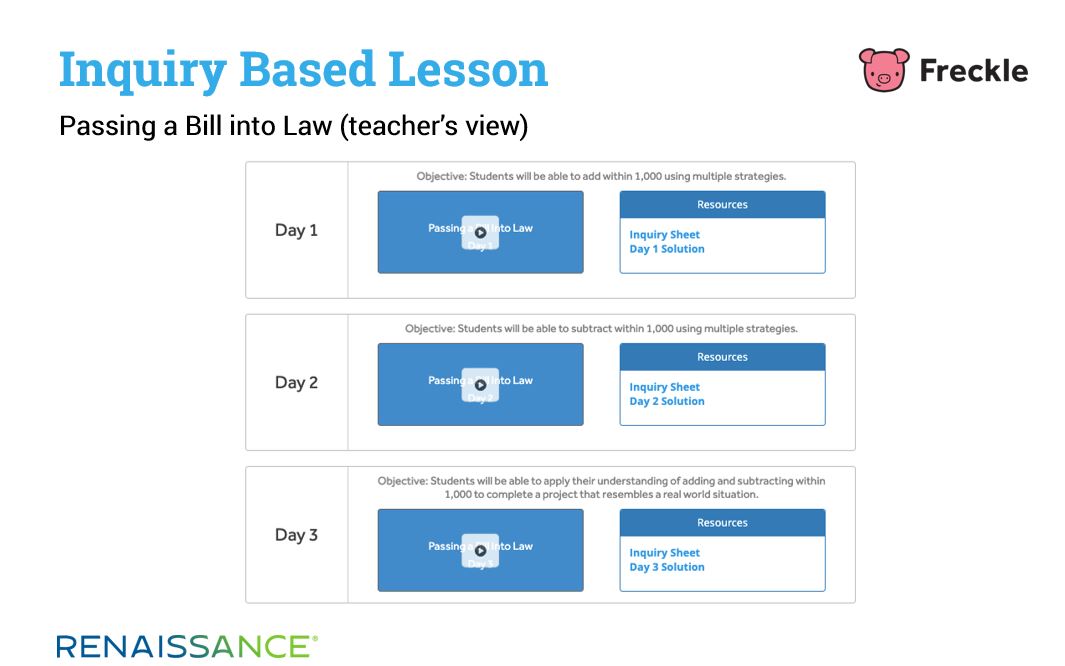
Summing it all up

In this blog, we’ve looked at math motivation through the lenses of executive function, emotional response, and the importance of a positive environment. We revisited Trip Steps for Mathematics, including the earliest Trip Step in pre-K, and found that executive function is critical in learning to count. We also identified the most difficult Trip Step (statistically speaking) in grade 3, and we examined an Algebra 1 Trip Step that can play a significant role in making public playgrounds more accessible.

As you prepare for the new school year, we invite you to explore the full list of Trip Steps—and to consider how focusing on executive function, emotional response, and math environment can be powerful motivators in your classroom this fall and beyond.

References

Barton, C. (2018). How I wish I’d taught maths. West Palm Beach: Learning Sciences International.
Cooper, A. (2017). How personalized learning starts with less teacher talk, more student voice. Retrieved from: https://www.edsurge.com/news/2017-01-25-how-personalized-learning-starts-with-less-teacher-talk-more-student-voice
Dehaene, S. (2011). The number sense: How the mind creates mathematics. Revised edition. New York: Oxford University Press.
Dehaene, S. (2017). A close look at the mathematician’s brain? Retrieved from: https://www.youtube.com/watch?v=EMAsQeLfr3o
Ellenberg, J. (2021). Want kids to learn math? Level with them that it’s hard. Retrieved from: https://www.washingtonpost.com/outlook/math-hard-easy-teaching-instruction/2021/06/25/4fbec7ac-d46b-11eb-ae54-515e2f63d37d_story.html
Hutchison, J., & Phillips, D. (n.d.). Supporting executive function during counting. Retrieved from: https://prek-math-te.stanford.edu/counting/supporting-executive-functioning-during-counting
Kroesbergen, E., Van Luit, J., Van Lieshout, E., Loosbroek, E., & Rijt, B. (2009). Individual differences in early numeracy: The role of executive functions and subitizing. Journal of Psychoeducational Assessment 27(3). http://dx.doi.org/10.1177/0734282908330586
Kimball, M., & Smith, N. (2013). There’s one key difference between kids who excel at math and those who don’t. Retrieved from: http://qz.com/139453/theres-one-key-difference-between-kids-who-excel-at-math-and-those-who-dont
November, A. (2018). Empowering teachers, engaging students. Education Week Webinar. Retrieved from: https://event.on24.com/wcc/r/1603764/20FBC8ECE6A4E7E1318B774D7167F7A4